Question

the cost of sandals has a mean of 18.30. if the z-score for a 23.70 pair of sandals is 1.8 what is the standard deviation?

Answer 1

The standard deviation of the cost of sandals is $2.50.

Step-by-step explanation:

The z-score is calculated using the formula:

`\[ z = ((X - \mu))/(\sigma) \]`

where
`\(X\)` is the value,
`\(\mu\)`is the mean, and
`\(\sigma\)` is the standard deviation. Given that the z-score for a $23.70 pair of sandals is 1.8, and the mean
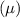`(\(\mu\))` is $18.30, we can rearrange the formula to solve for the standard deviation
`(\(\sigma\))`:

`\[ \sigma = ((X - \mu))/(z) \]`

Substituting the given values, we get:

`\[ \sigma = ((23.70 - 18.30))/(1.8) = (5.40)/(1.8) = 3.00 \]`

Therefore, the standard deviation is $3.00. It's essential to understand that the z-score represents how many standard deviations a data point is from the mean. In this case, a z-score of 1.8 indicates that the cost of the $23.70 sandals is 1.8 standard deviations above the mean.

The final step is to confirm this value by substituting the standard deviation back into the original z-score formula to ensure it accurately predicts the observed value:

`\[ z = ((X - \mu))/(\sigma) \]`

`\[ 1.8 = ((23.70 - 18.30))/(3.00) \]`

Solving for
`\(X\)`, we find
`\(X = 23.70\)`, confirming our calculation. Therefore, the standard deviation is $3.00.