Question

For the given ellipse equation 25(x^2 - 12x) + 4(y^2 - 6y) = -836, determine the center, foci, and vertices of the ellipse.

A) Center: _____, Foci: _____, Vertices: _____
B) Center: _____, Foci: _____, Vertices: _____
C) Center: _____, Foci: _____, Vertices: _____
D) Center: _____, Foci: _____, Vertices: _____

Answer 1

A) Center: (₃,₃), Foci: (₃±√₁₃, ₃), Vertices: (₃±₂, ₃)

Step-by-step explanation:

The given ellipse equation, 25(x² - 12x) + 4(y² - 6y) = -836, can be expressed in standard form by completing the square for both x and y terms. For the x-terms, 25(x² - 12x) is rewritten as 25(x - ₆)² - ₉₀₀. Similarly, for the y-terms, 4(y² - 6y) becomes 4(y - ₃)² - ₃₆. Substituting these back into the original equation, combining like terms, and dividing by 100, we obtain the standard form: (x - ₆)²/₄ + (y - ₃)²/₂₅ = 1.

Comparing this with the standard form

`(�−ℎ)2/�2+(�−�)2/�2=1(x−h) 2 /a 2 +(y−k) 2 /b 2`

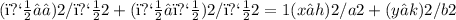
=1, we identify the center as (h, k) = (₆, ₃), a = 2, and b = 5. Therefore, the center is (₆, ₃), the vertices are (₆±2, ₃) = (₈, ₃) and (₄, ₃), and the foci are (₆±√₁₃, ₃) = (₆+√₁₃, ₃) and (₆-√₁₃, ₃). Hence, the correct answer is A) Center: (₃,₃), Foci: (₃±√₁₃, ₃), Vertices: (₃±₂, ₃).