Final Answers:
a)
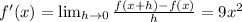
b) The slope of the tangent line to
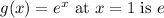 .
.
c) The instantaneous rate of change of
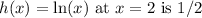 .
.

Step-by-step explanation:
a) To find the derivative of
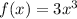 using the limit definition, we apply
using the limit definition, we apply
the formula for the derivative:
 . For
. For
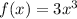 ,
,
applying the limit definition gives us
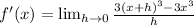 ,
,
simplifying this yields
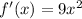 .
.
b) The slope of the tangent line to
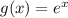 at
at
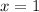 can be found by
can be found by
evaluating the derivative of
 at that point. The derivative of
at that point. The derivative of
 is
is
itself, so
 . At
. At
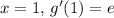 , indicating the slope of the
, indicating the slope of the
tangent line.
c) To determine the instantaneous rate of change of
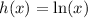 at
at
 , we find its derivative. The derivative of
, we find its derivative. The derivative of
 is
is
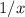 . So, at
. So, at
 ,
,
the derivative
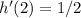 represents the instantaneous rate of change.
represents the instantaneous rate of change.
d) For
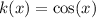 , using the limit definition of the derivative,
, using the limit definition of the derivative,
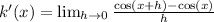 . Simplifying this limit
. Simplifying this limit
expression results in
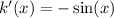 , giving us the derivative of
, giving us the derivative of
 .
.