Final Answer:
The complex number in trigonometric form is A)
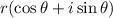 .
.
Explaination:
To represent a complex number in trigonometric form, we utilize Euler's formula:
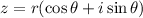 , where
, where
 is the complex number,
is the complex number,
 is the magnitude, and
is the magnitude, and
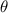 is the angle.
is the angle.
In this case, the form A)
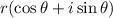 corresponds to the standard form of a complex number in trigonometric notation. The expression
corresponds to the standard form of a complex number in trigonometric notation. The expression
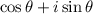 is derived from Euler's formula, which relates complex numbers to trigonometric functions. Here,
is derived from Euler's formula, which relates complex numbers to trigonometric functions. Here,
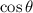 represents the real part of the complex number, and
represents the real part of the complex number, and
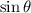 represents the imaginary part, combined with the angle
represents the imaginary part, combined with the angle
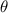 .
.
The use of
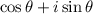 represents the magnitude
represents the magnitude
 along with the angle
along with the angle
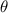 in the complex plane. It is a concise and standard way of expressing complex numbers, highlighting both magnitude and direction in terms of trigonometric functions, making it a convenient form for various mathematical operations and analyses involving complex numbers.
in the complex plane. It is a concise and standard way of expressing complex numbers, highlighting both magnitude and direction in terms of trigonometric functions, making it a convenient form for various mathematical operations and analyses involving complex numbers.