Question

A car drives horizontally off the edge of a cliff that is 35.7 m high. The police at the scene of the accident note that the point of impact is 146 m from the base of the cliff. How fast was the car traveling when it drove off the cliff?

Answer 1

The car was traveling at approximately 24.2 m/s when it drove off the cliff.

Step-by-step explanation:

To determine the car's initial velocity, we can use the kinematic equation for motion under gravity. When an object is in free fall, the vertical displacement can be related to the initial velocity, acceleration due to gravity, and time of flight. In this case, the vertical displacement is the height of the cliff (35.7 m), and the horizontal displacement is given (146 m).

Using the kinematic equation
`\(s = ut + (1)/(2)at^2\)`, where s is the vertical displacement, u is the initial velocity, a is the acceleration due to gravity, and t is the time of flight, we can solve for u. Since the car drives horizontally, the initial vertical velocity is zero.

Thus, we can ignore the ut term, leaving us with
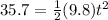`\(35.7 = (1)/(2)(9.8)t^2\)`. Solving for t and then using
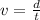`\(v = (d)/(t)\)` with the horizontal displacement d = 146, we find the initial velocity.