Final answer:
To find the exact sum of the series, we can use the formula for the sum of an infinite geometric series. The exact sum of the infinite geometric series 4 - 1 + 1/4 - 1/16 + 1/64 - 1/256 ... is 3.2.
Step-by-step explanation:
The given series is an infinite geometric series.
An infinite geometric series has the general form:
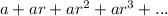
In this series, the first term 'a' is 4 and the common ratio 'r' is -1/4.
To find the exact sum of the series, we can use the formula for the sum of an infinite geometric series:
Sum = a / (1 - r)
Plugging in the values, we get:
Sum = 4 / (1 - (-1/4))
Simplifying, we get:
Sum = 4 / (1 + 1/4) = 4 / (5/4) = 16/5 = 3.2
Therefore, the exact sum of the infinite geometric series is 3.2.