Answer:
The company should not make 300 bicycles.
Explanation:
Mathematically speaking, profit (
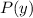 ) equals the difference between revenue (
) equals the difference between revenue (
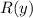 ) and cost (
) and cost (
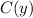 ). That is:
). That is:
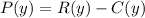 (1)
(1)
If we know that
 and
and
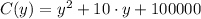 , then the profit function is:
, then the profit function is:
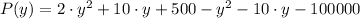
 (2)
(2)
The company can make the required amount of bicycles if and only if
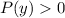 . If
. If
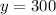 , then the profit of company is:
, then the profit of company is:
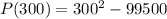
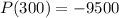
The company should not make 300 bicycles.