Question

Which of the following lines is parallel to the graph of 5x + 2y = 8?

Answer 1

From the analysis, the line in option (c)
`\(-10x-4y=7\)` is parallel to the graph of (5x+2y=8) as both have a slope of
`\(-(5)/(2)\).`

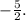
How to get the [parallel line

Let's check the slopes of the given equations:

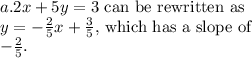
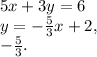
`a. \(2x+5y=3\) can be rewritten as \\\(y = -(2)/(5)x + (3)/(5)\), which has a slope of \\\(-(2)/(5)\).`

b.
`\(5x+3y=6\)\\ \(y = -(5)/(3)x + 2\),\\ \(-(5)/(3)\).`

c.
`\(-10x-4y=7\)\\ \(y = -(5)/(2)x - (7)/(4)\)\\ \(-(5)/(2)\).`

d.
`\(-10x+4y=9\) \\ \(y = (5)/(2)x + (9)/(4)\)\\ \((5)/(2)\).`

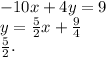
From the analysis, the line in option (c)
`\(-10x-4y=7\)` is parallel to the graph of (5x+2y=8) as both have a slope of
`\(-(5)/(2)\).`

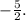
question

Which of the following lines is parallel to the graph of 5x + 2y = 8?Which of the following lines is parallel to the graph of 5x+2y=8

a. 2x+5y=3

b. 5x+3y=6

c. -10x-4y=7

d.-10x+4y=9