The value of the long division of 7·x³ + x²+ x by x² + 1 can be presented as follows;
(7·x³ + x² + x)/(x² + 1) =
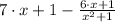
The steps used to divide (7·x³ + x² + x) by (x² + 1) using long division can be presented as follows;
7·x + 1
(x² + 1)|7·x³ + x² + x
7·x³ + 7·x
x² -6·x
x² + 1
-6·x -1
Therefore, (7·x³ + x² + x)/(x² + 1) is 7·x + 1, reminder -6·x - 1
Therefore, we get; (7·x³ + x² + x)/(x² + 1) = (7·x + 1) - (6·x + 1)/(x² + 1)