B =
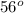
C =
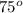
c = 38
The given question can be solved using the sine rule which states that;
 =
=
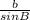 =
=
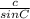
Given that;
A = 49 degrees, a = 30, and b = 33. Then.
 =
=
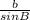
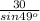 =
=
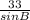
sin B = 0.8302
B =
 0.8302
0.8302
= 56.12
B =
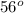
But,
A + B + C = 180
49 + 56 + C = 180
C = 180 - 105
= 75
C =
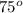
Then,
 =
=
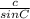
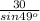 =
=
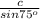
c = 38.396
c = 38