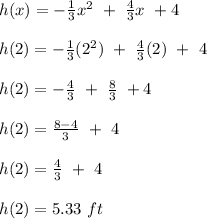Question

A frog leaps from a stump 4 feet high and lands 6 feet from the base of the stump. We can consider the initial position of the frog to be at (0, 4) and its landing position to be at (6, 0). It is determined that the height of the frog as a function of its horizontal distance x from the base of the stump is given by h(x) = -1/3x² + 4/3x + 4, where x and h(x) are both in feet. After how many feet did it reach its maximum height? What was the frog’s maximum height?

Answer 1

The time taken for the frog to reach maximum height is 2 seconds.

The maximum height reached by the frog is 5.33 feet.

How to calculate the time taken for the frog to reach maximum height?

The time taken for the frog to reach maximum height is calculated by applying the following formula as shown below.

`h(x) = - (1)/(3) x^2 \ + \ (4)/(3) x \ + 4\`

At maximum height, the final velocity of the frog = 0

v = d h(x) / dt

`v = - (2)/(3) x\ + \ (4)/(3)`

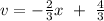
Recall that at maximum height, v = 0

0 = -2x/3 + 4/3

2x/3 = 4/3

Multiply both sides by 3

2x = 4

x = 4 / 2

x = 2 s

The maximum height reached by the frog is calculated as follows;

`h(x) = - (1)/(3) x^2 \ + \ (4)/(3) x \ + 4\\\\h(2) = - (1)/(3) (2^2) \ + \ (4 )/(3) (2) \ + \ 4\\\\ h(2) = - (4)/(3) \ + \ (8)/(3) \ + 4\\\\h(2) = (8 - 4)/(3) \ + \ 4\\\\h(2) = (4)/(3) \ + \ 4\\\\h(2) = 5.33 \ ft`