Final Answer:
Simplify form of the expression 9x ⁸ˣ −4x³ˣ is 4x³ˣ⁵ˣ(9x-1)³ˣ. Thus, the correct answer is C) 4x³ˣ⁵ˣ(9x-1)³ˣ.
Step-by-step explanation:
The expression
 can be factored by recognizing a common factor of
can be factored by recognizing a common factor of
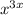 . Factoring out
. Factoring out
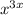 from both terms, we get:
from both terms, we get:
![\[x^(3x)(9x^(5x) - 4)\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/a7pu2hxzf023xwkylvaagtepsmeepvxzty.png)
Now, we can further factor the expression
 by recognizing a difference of squares pattern:
by recognizing a difference of squares pattern:
 . In this case, let
. In this case, let
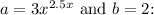
![\[x^(3x)[3x^(2.5x) + 2][3x^(2.5x) - 2]\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/g062okbz51ju3ed6wf2yn3vdp4yn25bwkd.png)
Now, notice that we have a common factor of
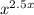 in both terms inside the brackets. Factor that out:
in both terms inside the brackets. Factor that out:
![\[x^(3x) \cdot 5x^(2.5x)(3x + 2)(3x - 2)\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/jblsr0aetxz46f65ze6vtnzaz6756fw9mz.png)
Finally, we can simplify
 , giving us the simplified expression:
, giving us the simplified expression:
![\[4x^(3x) \cdot 5x^(5x)(9x - 1)^(3x)\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/px0nh6i5zzljkzf3ylpw5sef6ok19r0wj4.png)
Therefore, the correct answer is C) 4x³ˣ⁵ˣ(9x-1)³ˣ.