For 7% interest compounded quarterly, monthly, and annually, the representations would be
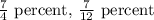 , and 7% respectively.
, and 7% respectively.
In the compound interest formula, the interest rate per period is represented as a fraction of the annual interest rate. If the interest rate is 7%, it would be represented as follows for different compounding periods:
1. Quarterly (4 times a year):
![\[ (7\%)/(4) \]](https://img.qammunity.org/2024/formulas/mathematics/college/svqmk44ry0fsce53sgsaxemdar8abj9cba.png)
So, for quarterly compounding, the interest rate per period would be
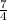 percent.
percent.
2. Monthly (12 times a year):
![\[ (7\%)/(12) \]](https://img.qammunity.org/2024/formulas/mathematics/college/8hpemi1iwkckqtab523qmgb7phlbph1xgy.png)
For monthly compounding, the interest rate per period would be
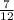 percent.
percent.
3. Annually (1 time a year):
![\[ (7\%)/(1) \]](https://img.qammunity.org/2024/formulas/mathematics/college/bs2anw2benvlvu0osznxxme0a53mlzto8a.png)
For annual compounding, the interest rate per period would be the full 7%.
Therefore, for 7% interest compounded quarterly, monthly, and annually, the representations would be
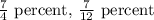 , and 7% respectively.
, and 7% respectively.
The probable question may be:
For example, if the interest rate is 3% compounded semiannually, this is represented in the compound interest formula as .03/2.
If the interest rate is 7% , how would that percent be represented in the compound interest formula if it was compounded
quarterly?
monthly?
annually?
Write answer as a fraction as above.