The linked answer is wrong because that integral gives you the net displacement of the object, not the total distance.
To get the distance, you have to integrate the speed (as opposed to velocity), which involves integrating the absolute value of the velocity function.
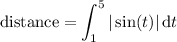
By definition of absolute value,
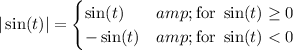
Over this particular integration interval,
• sin(t ) ≥ 0 for 1 ≤ t < π, and
• sin(t ) < 0 for π < t ≤ 5
so you end up splitting the integral at t = π as
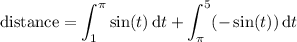
Now compute the distance:
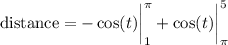
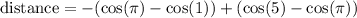
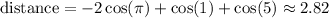
making B the correct answer.