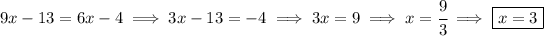Check the picture below.
First off let's notice that the angles at the vertex C are both equal, and those two triangles are right-triangles, and those two triangles share the hypotenuse Cw, thus we can say that both of those triangles at vertex C are congruent triangles by the "HA" theorem.
Now, let's take a look at the triangles touching the A vertex, △Axw and △Awz, both right-triangles and both also sharing the hypotenuse Aw and both with equal angles at A, so we can also say that both of those triangles are also congruent by the "HA" theorem.
What the hell all that means?
well, it means that yw = wx = wz = 9x - 13 = 6x - 4, even though the picture is quite misleading.