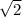The slant height of the largest possible cone that can be inserted in a hemisphere is c. 6
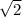
The volume of the hemisphere is given by:
Volume of hemisphere =

Given the volume as 144π
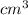
So, to set the equation,
144π = 2/3 * π *
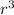
144π * 3 = 2 * π *
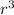
432π = 2π
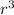
divide through by 2π
432π/2π = 2π
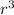 /2π
/2π
216 =
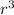
r = ∛216
r = 6
So, radius of the hemisphere = 6 cm
The slant height (l) of the cone can be found using the Pythagorean theorem in the right triangle formed by the radius (r), the height (h) of the cone.
So, l² = h² + r²
Where the height of the cone is the radius of the hemisphere,
l² = 2r²
l =
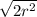
l =
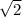 * r
* r
l =
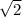 * 6
* 6
l = 6
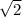
Therefore, the slant height of the largest possible cone that can be inserted in a hemisphere is c. 6