Question

Calculate the equilibrium concentration of Ni2+ in a 1.0-M solution [Ni(NH3)6] (NO3)2.

Answer 1

The equilibrium concentration of Ni2+ in a 1.0 M solution of [Ni(NH3)6] is
`\(2.0 * 10^8 \, \text{M}\)`, determined by the formation constant (Kf) expression and initial conditions.

To calculate the equilibrium concentration of Ni2+ in a 1.0 M solution of [Ni(NH3)6], we can use the formation constant (Kf) expression and the stoichiometry of the reaction:

`\[ \text{Ni}^(2+) + 6\text{NH}_3 \rightleftharpoons [\text{Ni(NH}_3)_6]^(2+) \]`

The formation constant expression is given as:

`\[ K_f = \frac{[\text{Ni(NH}_3)_6]^(2+)}{\text{[Ni}^(2+)][\text{NH}_3]^6} \]`

Given that
`\( K_f = 2.0 * 10^8 \)`, and initially, there is no
`\([\text{Ni(NH}_3)_6]^(2+)\)`, and the concentration of
`\(\text{NH}_3\)` is 1.0 M, we can set up the expression:

`\[ 2.0 * 10^8 = (x)/((1.0)^6) \]`

Solving for
`\(x\)`, the equilibrium concentration of
`\([\text{Ni(NH}_3)_6]^(2+)\)`:

`\[ x = 2.0 * 10^8 \, \text{M} \]`

Therefore, the equilibrium concentration of
`\(\text{Ni}^(2+)\)` in the solution is
`\(2.0 * 10^8 \, \text{M}\).`
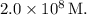
The probable question may be:

Calculate the equilibrium concentration of
`Ni2^+` in a 1.0 M solution
`[Ni(NH_3)_6]. Ni2+ (aq) + 6NH_3(aq) = [Ni(NH_3)_6]2^+(aq) Kf = 2.0 x 10^8`