Question

Give a short answer. I am looking for words such as convergent, divergent, not

enough information, ratio test, direct comparison test, etc.

Answer 1

The power series Σ
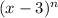`(x - 3)^n` has a radius of convergence of zero, indicating convergence only at x = 3. The interval of convergence is [3, 3], emphasizing that the series converges solely at x = 3 and diverges elsewhere.

The power series Σ
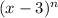`(x - 3)^n` has a radius of convergence of zero, indicating that the series converges only at the center, which is x = 3. The radius of convergence is the maximum distance from the center at which the series converges. In this case, it's zero, meaning the series converges only at x = 3 and nowhere else.

The interval of convergence is the range of x-values for which the series converges. Since the radius of convergence is zero, the interval of convergence is a single point: [3, 3]. This implies that the series converges only when x is exactly equal to 3 and diverges for all other values.

Understanding the radius and interval of convergence is crucial for determining where a power series converges and evaluating its behavior at different points. In this scenario, the power series is highly limited in its convergence, emphasizing the significance of these concepts in the study of power series and their applications in mathematics.