Question

Show work for the answer below

Answer 1

The correct answer is option B. cone, 150.7 cubic units

To determine the three-dimensional figure formed by rotating the triangle JKL about the line x = 2, we can imagine each point of the triangle tracing a circular path centered at x = 2. This rotation would generate a frustum of a cone.

The frustum of a cone is a three-dimensional figure that results from cutting a cone with a plane parallel to its base. In this case, the base of the cone is the original triangle JKL, and the cutting plane is perpendicular to the axis of rotation, x = 2.

To find the volume of the frustum of the cone, we can use the formula:

`\[ V = (1)/(3) \pi h (R^2 + r^2 + Rr) \]`

where
`\( R \)` and
`\( r \)` are the radii of the top and bottom circles of the frustum, and
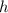`\( h \)` is the height of the frustum.

The height of the frustum is the horizontal distance between the axis of rotation and the top vertex of the original triangle, which is
`\( 6 - 2 = 4 \)` units.

The radii can be found using the distances between the vertices and the axis of rotation. The top radius
`\( R \)` is
`\( 6 - 2 = 4 \)` units, and the bottom radius
`\( r \)` is
`\( 2 - 2 = 0 \)` units.

Substituting these values into the formula:

`\[ V = (1)/(3) \pi \cdot 4 \cdot (4^2 + 0^2 + 4 \cdot 0) \]`

`\[ V = (1)/(3) \pi \cdot 4 \cdot 16 \]`

`\[ V = (64)/(3) \pi \]`

Using an approximate value for
`\( \pi \) (3.14)`, the volume is approximately 150.7 cubic units.

Therefore, the correct answer is:

B. cone, 150.7 cubic units