You would repay approximately $22,973.07 in total.
You would pay approximately $4,973.07 in interest over the 5 years.
How to solve
To calculate the total amount you would repay and the total interest paid on your loan, we can use the compound interest formula:
A =
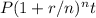
Where:
A is the total amount to be repaid
P is the principal loan amount ( $18,000)
r is the annual interest rate (5%)
n is the number of times interest is compounded per year (typically, once for annual compounding)
t is the time in years (5 years)
Step 1: Calculate the total amount to be repaid (A):
First, convert the interest rate from a percentage to a decimal: 5% / 100 = 0.05
Plug the values into the formula:
A = $18,000 * (1 + 0.05/1)^1*5
A ≈ $22,973.07
Therefore, you would repay a total of approximately $22,973.07.
Step 2: Calculate the total interest paid (I):
Subtract the principal amount from the total amount to find the total interest paid:
I = $22,973.07 - $18,000
I ≈ $4,973.07
Therefore, the total interest paid on the loan would be approximately $4,973.07.
So, to answer your questions:
You would repay approximately $22,973.07 in total.
You would pay approximately $4,973.07 in interest over the 5 years.
The Complete Question
Suppose you take out a loan of $18,000 from a financial institution with an annual interest rate of 5% compounded annually. Over a period of 5 years, how much total money (loan amount + interest) would you repay, and what would be the total interest paid on the loan?