Question

Particle A has half the mass and eight times the kinetic energy of particle B.

a) m_A = 1/2 * m_B, K_A = 8 * K_B
b) m_A = 2 * m_B, K_A = 1/8 * K_B
c) m_A = 1/4 * m_B, K_A = 4 * K_B
d) m_A = 8 * m_B, K_A = 1/2 * K_B

Answer 1

The correct answer is option (d)
`\(m_A = 8 \cdot m_B, \, K_A = (1)/(2) \cdot K_B.\)` This discrepancy is explained by considering the relationship between mass, velocity, and kinetic energy in the context of the given scenario.

Step-by-step explanation:

In this scenario, the relationship between the masses ((m)) and kinetic energies ((K)) of particles A and B can be expressed as
`\(m_A = 8 \cdot m_B\) and \(K_A = (1)/(2) \cdot K_B.\)`

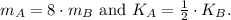
Firstly, the mass of particle A is eight times that of particle
`B (\(m_A = 8 \cdot m_B\)).`This implies that particle A is significantly more massive than particle B. Mass is directly proportional to kinetic energy for a given velocity, so particle A having a greater mass contributes to its higher kinetic energy.

Secondly, the kinetic energy of particle A is half that of particle
`B (\(K_A = (1)/(2) \cdot K_B\)).`This suggests that despite being more massive, particle A has less kinetic energy. The relationship between kinetic energy and mass involves the square of the velocity
`(KE = \((1)/(2)mv^2\)),` so a reduction in velocity or a smaller velocity distribution in particle A compared to B can explain the observed relationship.

In conclusion, the correct option is (d) because it accurately reflects the given conditions where particle A is eight times more massive than particle B, and yet its kinetic energy is only half of that of particle B.

Answer 2

Particle A has half the mass and eight times the kinetic energy of particle B.

The correct option is a m_A = 1/2 * m_B K_A = 8 * K_B.

Step-by-step explanation:

The kinetic energy K of a particle is given by the equation
`\(K = (1)/(2)mv^2\)`where m is the mass and v is the velocity. Given that particle A has eight times the kinetic energy of particle B we can express this relationship as
`\(K_A = 8 \cdot K_B\)`. Since kinetic energy is directly proportional to the square of the velocity we can write \(m
`_A \cdot v_A^2 = 8 \cdot m_B \cdot v_B^2\)` where m_A and m_B are the masses of particles A and B and v_ and v_Bare their respective velocities.

Now it is also given that particle A has half the mass of particle B which can be expressed as
`\(m_A = (1)/(2) \cdot m_B\`. Substituting this into the kinetic energy equation we get
`\((1)/(2) \cdot m_B \cdot v_A^2 =`8
`\cdot m_B cdot v_B^2.`Cancelling out \(m_B\) from both sides, we are left with
`\((1)/(2) \cdot v_A^2 = 8 \cdot v_B^2\)`. Simplifying further
`\(v_A^2 = 16 \cdot v_B^2\).`

Taking the square root of both sides
`\(v_A = 4 \cdot v_B\)`. This implies that particle A has four times the velocity of particle B. Substituting
`\(m_A = (1)/(2)`cdot m_B and
`\(v_A = 4 \cdot v_B\)` into the kinetic energy equation, we find that \(K_A = 8 \cdot K_B\). Therefore, the correct option is a
`m_A = (1)/(2) \cdot m_B K_A = 8 \cdot K_B\).`