Final answer:
To verify if Rolle's Theorem applies to a square root function, we need to ensure the function is continuous and differentiable on a certain interval and has equal values at endpoints. Without specific information about the function and interval, we cannot confirm Rolle's Theorem's applicability so the answer is 'Insufficient information.'
Step-by-step explanation:
To verify that the function satisfies Rolle's Theorem for the square root, we need to consider the criteria for Rolle's Theorem which are:
- The function must be continuous on the closed interval
![[a, b]](https://img.qammunity.org/2024/formulas/mathematics/high-school/qx51vpjanvcbo50vxina1hdjwby271575g.png) .
. - The function must be differentiable on the open interval
 .
. - The function must have equal values at the endpoints of the interval, which means
 .
.
Given that the function in question involves a square root, we must ensure that the square root function satisfies all these conditions for some interval
![[a, b]](https://img.qammunity.org/2024/formulas/mathematics/high-school/qx51vpjanvcbo50vxina1hdjwby271575g.png) .
.
If, for example the function is
 , and the interval is
, and the interval is
![[0, 4]](https://img.qammunity.org/2024/formulas/mathematics/high-school/38tr01rxc78kimi05wnbqrac4w9gb2y416.png) , we know that:
, we know that:
-
 is continuous on
is continuous on
![[0, 4]](https://img.qammunity.org/2024/formulas/mathematics/high-school/38tr01rxc78kimi05wnbqrac4w9gb2y416.png) .
. -
 is differentiable on
is differentiable on
 but not at
but not at
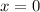 because the derivative of
because the derivative of
 , which is undefined at
, which is undefined at
 .
. -
 and
and
 , which shows that
, which shows that
 .
.
Therefore, without more specific information about the function and the interval, we cannot confirm whether Rolle's Theorem applies. As such, the correct answer is c) Insufficient information.