Question

Given the inequality tan(x)<0 and cos(x)<0, in which quadrant or quadrants does the angle x lie?

a) Quadrant I
b) Quadrant II
c) Quadrant III
d) Quadrant IV

Answer 1

The angle x must lie in Quadrant II where the sine function is positive and the cosine function is negative which leads to a negative value for the tangent function.

Step-by-step explanation:

The inequality
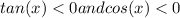`tan(x) < 0 and cos(x) < 0` indicates that x must lie in a quadrant where the tangent function is negative and the cosine function is also negative. The tangent function is negative where the sine and cosine functions have opposite signs. Since the cosine is negative as given in the inequality we must find a quadrant where sine is positive and cosine is negative for the tangent (which is sine divided by cosine) to be negative.

Recall that in the unit circle:

• In Quadrant I, both sine and cosine are positive.
• In Quadrant II, sine is positive and cosine is negative.
• Quadrant III, sine is negative and cosine is negative.
• In Quadrant IV, sine is negative and cosine is positive.

Hence, the angle x must lie in Quadrant II where sine is positive and cosine is negative, resulting in a negative tangent value.