Question

The Lunatik is a military spacecraft on extended patrol in the vicinity of the planet Eqrgp and its moon, with the mission of

repelling Vegan invaders who threaten life on Eqrgp or on its moon. The Vegans' home base is on a planet of the star Vega. As it
happens, Vegans are vegans, but still very vicious toward non-Vegan life forms, especially non-vegan ones. The captain of the
Lunatik endangers his craft's vital mission by running out of fuel, leaving the Lunatik adrift in space, subject to the gravitational
pulls of Eqrgp and its moon. Ignore the gravitational pulls of other bodies.
The Lunatik's navigator notices that for a short while the craft does not change its speed or direction. How far from the center of
Eqrgp is the Lunatik at that time? The distance between the centers of Eqrgp and its moon is 3.57 x 108 m. The mass of Egrap
is 5.87 x 1024 kg and the mass of its moon is 1.63 x 1023 kg.

Answer 1

The Lunatik is approximately 3.40 x 10^8 meters from the center of Eqrgp when it momentarily does not change speed or direction due to the gravitational equilibrium point.

To determine the distance from the center of Eqrgp to the Lunatik when it momentarily does not change speed or direction, we can use the concept of the gravitational equilibrium point between the planet and its moon. This point is where the gravitational forces from the planet and the moon balance out.

The gravitational force between Eqrgp and the Lunatik is given by Newton's law of gravitation:

`\[ F_{\text{planet}} = \frac{G \cdot M_{\text{planet}} \cdot m_{\text{Lunatik}}}{r_{\text{planet}}^2} \]`

The gravitational force between the moon and the Lunatik is:

`\[ F_{\text{moon}} = \frac{G \cdot M_{\text{moon}} \cdot m_{\text{Lunatik}}}{r_{\text{moon}}^2} \]`

At the gravitational equilibrium point, these forces are equal:

`\[ F_{\text{planet}} = F_{\text{moon}} \]`

Setting the two expressions equal to each other and solving for the distance
`\(r_{\text{planet}}\)` from the center of Eqrgp:

`\[ \frac{G \cdot M_{\text{planet}} \cdot m_{\text{Lunatik}}}{r_{\text{planet}}^2} = \frac{G \cdot M_{\text{moon}} \cdot m_{\text{Lunatik}}}{(d - r_{\text{planet}})^2} \]`

Where d is the distance between the centers of Eqrgp and its moon.

Simplify and solve for
`\(r_{\text{planet}}\):`

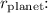
`\[ r_{\text{planet}} = \frac{d \cdot M_{\text{planet}}}{M_{\text{planet}} + M_{\text{moon}}} \]`

Substitute the given values:

`\[ r_{\text{planet}} = \frac{(3.57 * 10^8 \, \text{m}) \cdot (5.87 * 10^(24) \, \text{kg})}{(5.87 * 10^(24) \, \text{kg}) + (1.63 * 10^(23) \, \text{kg})} \]`

Calculate the result:

`\[ r_{\text{planet}} \approx 3.40 * 10^8 \, \text{m} \]`