Final answer:
The correct asymptotic order of growth for the function log
 as the √n term dominates the log n term for large values of n.
as the √n term dominates the log n term for large values of n.
Step-by-step explanation:
To determine the asymptotic order of growth of the function log
 we should consider the behavior of each term separately as n approaches infinity. The natural logarithm function grows slower than the square root function. Therefore, as n gets very large, the
we should consider the behavior of each term separately as n approaches infinity. The natural logarithm function grows slower than the square root function. Therefore, as n gets very large, the
 term will dominate, and the log n term will become relatively insignificant in comparison. Thus, the function log
term will dominate, and the log n term will become relatively insignificant in comparison. Thus, the function log
 will behave similarly to just
will behave similarly to just
 for large values of n.
for large values of n.
Comparing the options given, the correct inequality that describes the asymptotic order of growth for
 is:
is:
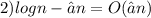
This is because as n becomes very large, the negative square root term will dictate the growth behavior of the function, and thus, the function is bounded by a constant multiple of
 , satisfying the definition of Big O notation.
, satisfying the definition of Big O notation.