Question

Select the correct answer.

The graph shows an outer square which is parallel to the axis and starts from the origin (0, 0) and ends point intercepts the x-axis at (a, 0), And the inner triangle intercepts the x-axis at (a minus b, 0) and the y-axis at (0, b).

What is the ratio of the area of the inner square to the area of the outer square?

A.
B.
C.

Answer 1

The ratio of the area of the inner square to the area of the outer square is found by dividing the area of the inner square,
`(a-b)^2`, by the area of the outer square,
`a^2`, resulting in the ratio (
`(a-b)^2)/(a^2).`

To find the ratio of the area of the inner square to the area of the outer square when given specific intercepts on the axes, we first need to determine the side lengths of both squares. The outer square has one vertex at the origin (0,0) and another at (a, 0), so its side length is a. The area of the outer square is therefore a squared, or
`a^2.`

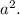
The inner triangle has x-axis intercepts at (a-b, 0), meaning the side of the inner triangle is a-b. Assuming this triangle is actually meant to be an inner square as per the question's main focus, since triangles do not have area ratios to squares, its area would be
`(a-b)^2.` To find the ratio of the area of the inner square to the outer square, we divide the area of the inner square by the area of the outer square:
`((a-b)^2) / (a^2).`

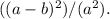
This result expresses the ratio of the inner square's area to the outer square's area in terms of a and b, which are based on the intercepts given on the x-axis and y-axis.