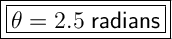Question

Find the central angle .

Answer 1

2.5 rad

Explanation:

`l=r\alpha\Rightarrow\alpha=(l)/(r)=(10)/(4)rad=2.5rad`

Answer 2

2.5

Explanation:

The find the measure of the central angle, we can use the arc length formula:

`\boxed{\begin{array}{l}\underline{\sf Arc\; length}\\\\s=r \theta\\\\\textsf{where:}\\\phantom{ww}\bullet\; \textsf{$s$ is the arc length.}\\ \phantom{ww}\bullet\; \textsf{$r$ is the radius.}\\ \phantom{ww}\bullet\;\textsf{$\theta$ is the angle measured in radians.}\end{array}}`

In this case:

• s = 10
• r = 4

Substitute the values of sS and r into the formula, then solve for θ:

`\begin{aligned}10&=4 \cdot \theta\\\\\theta&=(10)/(4)\\\\\theta&=2.5\end{aligned}`

Therefore, the radian measure of an angle θ, where s is the length of the circular arc that subtends θ in a circle of radius r is:

`\Large\boxed{\boxed{\theta=2.5\; \sf radians}}`