Final Answer:
The expression (9x²y² ,dx + 6x³y ,dy) is an exact differential.
Step-by-step explanation:
To determine if the given expression is an exact differential, we need to check if the mixed partial derivatives with respect to (x) and (y) are equal. The expression is an exact differential if and only if:
![\[ (\partial)/(\partial y)(9x^2y^2) = (\partial)/(\partial x)(6x^3y) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/zvozgduqvym1w0yyhnvzwwp49tvlfanupu.png)
Let's calculate the partial derivatives:
1.
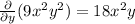
2.

Since
 , the expression is an exact differential.
, the expression is an exact differential.
Now, we can find the potential function \(U(x, y)\) by integrating the given expression with respect to \(x\) and then integrating the result with respect to \(y\):
![\[ U(x, y) = \int (9x^2y^2 \,dx + 6x^3y \,dy) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ju9j7w56u2iqlsnlrt7o5nt7wk7u25e1lg.png)
Integrating the first term with respect to (x) gives (3x³y² + C(y)), where (C(y)) is a constant of integration. Now, integrating the result with respect to (y) gives:
![\[ U(x, y) = x^3y^3 + C(y) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/knnslsvvjerrl15ur76g6lmp1xlfp8uh60.png)
Thus, the potential function
 confirms that
confirms that
 is indeed an exact differential.
is indeed an exact differential.