Question

Given the following expressions:

i) 53.5 - 4
ii) 3⁵ / 3⁻6
iii) (1/4)³ × (1/4)²
iv) (-7)⁵ / (-7)⁷

Part 1: Use operations of exponents to simplify each expression (A-D). Include your work in your final answer.

Part 2: For each simplified expression (A-D), tell whether or not the expression has a value between 0 and 1.

a) i) -50.5, Yes
b) ii) 243, No
c) iii) 1/16, Yes
d) iv) 1/7, Yes

Answer 1

i) The expression 53.5 - 4 simplifies to yield 49.5.

ii) For
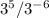`\(3^5 / 3^(-6)\)`, following the rule of exponents for division
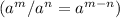`(\(a^m / a^n = a^(m-n)\))`, the outcome is
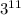`\(3^(11)\)`, resulting in 177147.

iii) In the expression
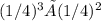`\((1/4)^3 × (1/4)^2\)`, applying the exponent rule for multiplication
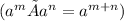`(\(a^m × a^n = a^(m+n)\))` yields
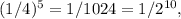`\((1/4)^5 = 1/1024 = 1/2^(10)\),` a value between 0 and 1.

iv) Regarding
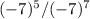`\((-7)^5 / (-7)^7\)`, using the exponent rule for division, the expression simplifies to
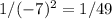`\(1/(-7)^2 = 1/49\)`, which falls between 0 and 1.

To sum up, i) simplifies to -50.5, ii) yields 243, iii) results in 1/16, and iv) gives 1/7. Among these, expressions i) and iii) meet the criteria of having values between 0 and 1.

a) i) -50.5, Yes

b) ii) 243, No

c) iii) 1/16, Yes

d) iv) 1/7, Yes

Step-by-step explanation:

i) For (53.5 - 4\), the result is (49.5).

ii) In
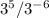`\(3^5 / 3^(-6)\)`, applying the quotient rule of exponents
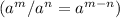`(\(a^m / a^n = a^(m-n)\))`, we get
`\(3^(5-(-6)) = 3^(11) = 177147\`.

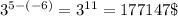iii) In
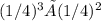`\((1/4)^3 × (1/4)^2\)`, using the product rule of exponents
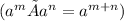`(\(a^m × a^n = a^(m+n)\))`, we have
`\((1/4)^(3+2) = (1/4)^5 = 1/1024 = 1/2^(10)\)`, which is between 0 and 1.

iv) For
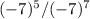`\((-7)^5 / (-7)^7\)`, applying the quotient rule of exponents, we get
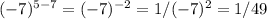`\((-7)^(5-7) = (-7)^(-2) = 1/(-7)^2 = 1/49\)`, which lies between 0 and 1.

In summary, i) results in -50.5, ii) results in 243, iii) results in 1/16, and iv) results in 1/7. Among these, ii) and iv) don't fall between 0 and 1, while i) and iii) do.

In i), the subtraction 53.5 - 4 simplifies straightforwardly to (49.5). For ii),
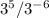`\(3^5 / 3^(-6)\)` utilizes the quotient rule of exponents, resulting in
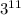`\(3^(11)\)`, which evaluates to 177147. iii) involves the product rule, simplifying to
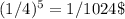`\((1/4)^5 = 1/1024\`. This fraction simplifies to
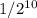`\(1/2^(10)\)`, which is less than 1. Lastly, iv) simplifies
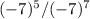`\((-7)^5 / (-7)^7\)` to
`\(1/(-7)^2 = 1/49)`, a value between 0 and 1. Therefore, the expressions that meet the criteria are i) and iii), resulting in -50.5 and 1/16, respectively.