Final Answer:
The equivalent expressions among the given options are:
A)
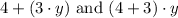
Step-by-step explanation:
In the provided options, we need to identify equivalent expressions. Looking at option A, we have
 and \((4 + 3) \cdot y\). To check their equivalence, let's simplify both expressions.
and \((4 + 3) \cdot y\). To check their equivalence, let's simplify both expressions.
For
 , we follow the order of operations (PEMDAS/BODMAS), which stands for Parentheses/Brackets, Exponents/Orders, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). Here, we first perform the multiplication inside the parentheses:
, we follow the order of operations (PEMDAS/BODMAS), which stands for Parentheses/Brackets, Exponents/Orders, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). Here, we first perform the multiplication inside the parentheses:
![\[4 + (3 \cdot y) = 4 + 3y\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/v5ixfb37k13gyfgjfuuw3wvor2r3codch7.png)
Now, let's simplify the expression on the right side of option A,
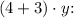
![\[(4 + 3) \cdot y = 7 \cdot y\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/bed4gjznds6rvhxsne0u6q30m3pjug37hi.png)
Comparing the two simplified expressions,
 and (7y), we can see they are not equivalent. Therefore, option A does not contain equivalent expressions. The correct answer is
and (7y), we can see they are not equivalent. Therefore, option A does not contain equivalent expressions. The correct answer is
 and
and
 .
.