Main Answer:
The positive difference between
 is 64 (Option B).
is 64 (Option B).
Therefore, the correct answer is (Option B).
Step-by-step explanation:
The positive difference between two exponents can be calculated by subtracting the smaller exponent from the larger one. In this case, we subtract
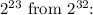
![\[2^(32) - 2^(23) = 2^(23)(2^9 - 1)\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ruzjt9mz7s9q3w6e00sbq39kj4k00gqatv.png)
Now,
 and subtracting 1 gives us 511. So, the positive difference is
and subtracting 1 gives us 511. So, the positive difference is
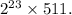 To find this value, we can use the fact that
To find this value, we can use the fact that
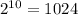 and then adjust for the
and then adjust for the
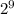 factor:
factor:
![\[2^(23) * 511 = 2^(23) * (2^(10) - 1) = 2^(23) * 1023\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/qtwq058zpxapw631ikjk40p8lerv0m1ibw.png)
Now,
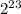 is a common factor, so we can express the positive difference as
is a common factor, so we can express the positive difference as
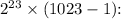
![\[2^(23) * 1022\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/6yyt7ww96pbym42b3y9mgxrctqdvdibjwt.png)
Since
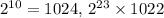 is essentially
is essentially
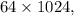 and that equals 65536. Therefore, the positive difference between
and that equals 65536. Therefore, the positive difference between
 is indeed 64.
is indeed 64.
Therefore, the correct answer is (Option B).