Question

What is the particle's speed at d ? express your answer with the appropriate units.

Answer 1

The speed of a particle is the magnitude of the velocity vector. As an example, given the velocity function

`v(t) = 3.0 + 1.5t² m/s`, the speed at
`t = 2.0 s` would be calculated as
`9.0 m/s`. It's crucial to provide the units which, in this context, are meters per second (m/s).

Step-by-step explanation:

To determine the speed of a particle at distance d, you need to have a specific function that relates speed, distance, or time. In general, speed is a scalar quantity that represents how fast a particle is moving, regardless of its direction of travel. It is the magnitude of the velocity vector and is given by the equation:

`Speed = |v(t)|`

For instance, given a velocity function
`v(t) = 3.0 + 1.5t² m/s`, you can calculate the speed at a specific time by taking the absolute value of the velocity. Substituting t = 2.0 s into the equation:

`v(2.0 s) = 3.0 + 1.5(2.0)² m/s = 9.0 m/s`

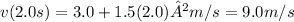
Thus, the speed of the particle at
`t = 2.0 s is 9.0 m/s.` It's important to express the answer with the appropriate units, which in this case is meters per second (m/s). Similarly, for other given functions or scenarios, you follow the same process to calculate the speed at a particular instance based on the provided formula for velocity or position as a function of time.