The margin of error for a 95% confidence interval for the population mean is approximately 5.35 (rounded to three decimal places).
The margin of error for a 95% confidence interval for the population mean
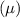 can be calculated using the formula:
can be calculated using the formula:
![\[ \text{Margin of Error} = Z * (\sigma)/(√(n)) \]](https://img.qammunity.org/2024/formulas/mathematics/college/aiuiopuea5e6xg2kr4xkcilhh10zemsc36.png)
Where:
 is the Z-score corresponding to the desired confidence level (for a 95% confidence interval,
is the Z-score corresponding to the desired confidence level (for a 95% confidence interval,
 ,
,
σ is the population standard deviation,
 is the sample size.
is the sample size.
Let's plug in the values:
![\[ \text{Margin of Error} = 1.96 * (24)/(√(77)) \]](https://img.qammunity.org/2024/formulas/mathematics/college/fkhacegfro42humlotn4l259l2zg9wm653.png)
Now, let's calculate it:
![\[ \text{Margin of Error} \approx 1.96 * (24)/(√(77)) \]](https://img.qammunity.org/2024/formulas/mathematics/college/x77g6ul8m1mcwhmm64skuu7ckor45irtc5.png)
![\[ \text{Margin of Error} \approx 1.96 * (24)/(8.775) \]](https://img.qammunity.org/2024/formulas/mathematics/college/uvbs8wsl1dd3di8akvl1nyw7m38saj3aah.png)
![\[ \text{Margin of Error} \approx 1.96 * 2.735 \]](https://img.qammunity.org/2024/formulas/mathematics/college/wmcwcuk831z10edhugolj82g7sb16fwzfz.png)
![\[ \text{Margin of Error} \approx 5.35 \]](https://img.qammunity.org/2024/formulas/mathematics/college/627rchzq0sho9ti90m02ed81faesrzrs1r.png)
Therefore, the margin of error for a 95% confidence interval for the population mean is approximately 5.35 (rounded to three decimal places).