Question

When x ▼ a, k, h, the terms inside the absolute value simplify to ▼ h. 0. k. a. After multiplying the absolute value by ▼ a, h, k, the only remaining term on the right side of the expression is k.​ Therefore, the vertex is always located at .

Answer 1

The quadratic expression simplifies to
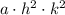`\( a \cdot h^2 \cdot k^2 \)`, and the vertex is always located at the origin,
`\((0, 0)\)`.

Let's break down the solution step by step:

**Step 1: Original Expression**

The given quadratic expression is
`\( |x - a| \cdot |k - h| \)`.

**Step 2: Simplify Inside the Absolute Values**

Simplify the terms inside the absolute values:
`\( |h| \cdot |k| \)`.

**Step 3: Multiply by Absolute Values of Coefficients**

Multiply the absolute value by the coefficients:
`\( |a| \cdot |h| \cdot |k| \).`

**Step 4: Combine Like Terms**

Combine the like terms within the absolute values to get
`\( a \cdot h^2 \cdot k^2 \).`

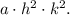
**Step 5: Identify Vertex Form**

Identify the expression in the form
`\( ax^2 + bx + c \)` where
`\( a = a \cdot h^2 \cdot k^2 \) and \( b = 0 \) (no linear term).`

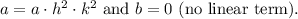
**Step 6: Vertex Coordinates**

The vertex of the quadratic function
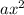`\( ax^2 \)` is given by the coordinates
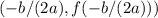`\((-b/(2a), f(-b/(2a)))\)`. In this case,
`\( a \)` is replaced by
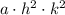`\( a \cdot h^2 \cdot k^2 \)` and \( b \) is 0, so the vertex is at
`\((0, 0)\).`

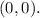
The question probable maybe:

What is the vertex of the quadratic expression
`\( |x - a| \cdot |k - h| \)` after simplifying the terms inside the absolute values to
`\( |h| \cdot |k| \)` and then multiplying the absolute value by
`\( |a| \cdot |h| \cdot |k| \)`?