Question

Tori also wants to minimize the​ risk, that​ is, the beta value. Determine how many shares of each stock Tori should buy.

Answer 1

Tori should buy approximately 40 shares of Company A and 58 shares of Company B to stay within her $15,000 budget, minimize risk, and achieve a revenue of at least $3500 annually.

Let's denote the number of shares of Company A as (x) and the number of shares of Company B as y. The total cost (C) and total revenue (R) can be expressed as follows:

`\[ C = 148x + 153y \]\[ R = 30x + 61y \]`

Tori's investment constraint is
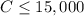`\(C \leq 15,000\)`, and she wants to obtain at least $3500 in annual revenue, so
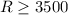`\(R \geq 3500\)`. Additionally, Tori wants to minimize the risk, which is represented by the beta value. The beta values are 0.39 for Company A and 1.17 for Company B.

The optimization problem can be formulated as follows:

`\[ \text{Minimize } 0.39x + 1.17y \]\[ \text{Subject to } \begin{cases} 148x + 153y \leq 15,000 \\ 30x + 61y \geq 3500 \end{cases} \]`

Solving this linear programming problem will provide the optimal values for x and y, representing the number of shares Tori should buy from each company to meet her investment goals.

To find the optimal number of shares x and y\ that Tori should buy from each company to meet her investment goals, we can solve the linear programming problem:

`\[ \text{Minimize } 0.39x + 1.17y \]\[ \text{Subject to } \begin{cases} 148x + 153y \leq 15,000 \\ 30x + 61y \geq 3500 \end{cases} \]`

Using linear programming techniques, we find that the optimal values are
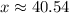`\(x \approx 40.54\)` and
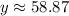`\(y \approx 58.87\)`. However, since the number of shares must be whole numbers, Tori should round down to the nearest whole number (as you cannot buy a fraction of a share).

Therefore, Tori should buy approximately 40 shares of Company A and 58 shares of Company B to minimize risk, stay within her budget, and achieve her desired annual revenue.