Question

Geometry, Finding angles. Images of the problem attatched below

Answer 1

Check the picture below.

keep in mind that twin sides make twin angles, and an angle bisector from B makes a perpendicular with the base.

`6x-9=4x+7\implies 2x-9=7\implies 2x=16\implies x=\cfrac{16}{2}\implies x=8 \\\\[-0.35em] ~\dotfill\\\\ AC= 5x-12\implies AC=5(8)-12\implies AC=28`

now, for the area of the triangle, well, let's keep in mind it has a base of AC and a height of BD, hmmm what's BD anyway?

`\stackrel{base}{AC=28}\hspace{5em}AD=\cfrac{AC}{2}\implies AD=14 \\\\\\ AB=6x-9\implies AB=6(8)-9\implies AB=39 \\\\[-0.35em] ~\dotfill\\\\ \begin{array}{llll} \textit{using the pythagorean theorem} \\\\ AD^2+BD^2=AB^2\implies BD=√(AB^2 - AD^2) \end{array} \qquad \begin{cases} AB=\stackrel{hypotenuse}{39}\\ AD=\stackrel{adjacent}{14}\\ BD=opposite \end{cases} \\\\\\ BD=√( 39^2 - 14^2)\implies BD=√( 1521 - 196 ) \implies BD=√( 1325 ) \\\\[-0.35em] ~\dotfill`

`\stackrel{\textit{\\ormalsize so we can say, what's the area of a triangle with b=14 and h=}√(1325)?}{Area=\cfrac{1}{2}(\underset{b}{14})(\underset{h}{√(1325))}\implies Area\approx 254.8}`

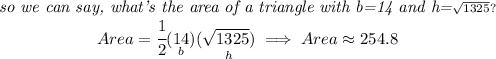
Now, if ∡A = 7x or ∡A = 56 = ∡C, that makes ∡B= 180 - 56 - 56 = 68°.