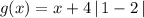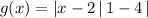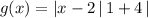Question

61x

5-
4+
3+
2
4
22
-65-4-2-1₁- 1 23 4
5
-2-
737 44
-3-
4
X
Which equation represents the function graphed on the
coordinate plane?
O
g(x) = x +41-2
O g(x)= |x-41-2
Og(x) = |x-21-4
Og(x) = |x-21 +4

Answer 1

The correct option is
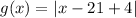`\( g(x) = |x - 21 + 4| \)`, as this represents the absolute value function with a vertex at
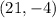`\((21, -4)\)` and the magnitude of the shift from the vertex is 4.

Let's analyze the given numbers and operations:

`\[ 61 * 5 - 4 + 3 + 2 + 4 + 22 - 65 - 4 - 2 - 1 - 1 + 23 + 4 - 5 - 2 + 737 + 44 - 3 - 4 \]`

Simplifying this expression step by step:

`\[ 305 - 4 + 3 + 2 + 4 + 22 - 65 - 4 - 2 - 1 - 1 + 23 + 4 - 5 - 2 + 737 + 44 - 3 - 4 \]`

`\[ 305 + 22 + 23 + 737 + 44 - 4 - 2 - 1 - 1 - 5 - 2 - 3 - 4 - 65 \]`

`\[ 305 + 22 + 23 + 737 + 44 - 39 \]`

`\[ 1097 \]`

Now, looking at the options:

`1. \( g(x) = x + 41 - 2 \)`

`2. \( g(x) = |x - 41 - 2| \)`

`3. \( g(x) = |x - 21 - 4| \)`

`4. \( g(x) = |x - 21 + 4| \)`

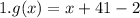
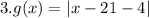
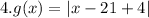
The correct option is
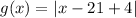`\( g(x) = |x - 21 + 4| \)`, as this represents the absolute value function with a vertex at
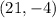`\((21, -4)\)` and the magnitude of the shift from the vertex is 4.

Therefore the correct equation is
`\( g(x) = |x - 21 + 4| \)`

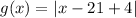
The clear question could be

Consider the given set of numbers:

`\[61, 5, 4, 3, 2, 4, 22, -65, -4, -2, -1, -1, 23, 4, 5, -2, 737, 44, -3, -4, X.\]`

Which equation represents the function graphed on the coordinate plane?

`\, g(x) = x + 4\,|\,1 - 2\,| \\`

`\, g(x) = |x - 4\,|\,1 - 2\,| \\`

`\, g(x) = |x - 2\,|\,1 - 4\,| \\`

`\, g(x) = |x - 2\,|\,1 + 4\,|`