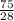Question

\frac{-x+3}{4}+15=6\left(x-3\right)+15

Answer 1

`x = (75)/(28)`

Explanation:

-x + 3/4 + 15 = 6(x-3) + 15

Add:

-x + 3/4 + 15 = 6(x-3) + 15

-x + 63/4 = 6 (x-3) + 15

Distribute:

-x + 63/4 = 6(x-3) + 15

-x + 63/4 = 6x - 18 + 15

Add:

-x + 63/4 = 6x - 18 + 15

-x + 63/4 = 6x-3

-x + 63/4 = 6x - 3

Subtract 63/4 from both sides:

-x + 63/4 - 63/4 = 6x - 3 - 63/4

Subtract:

-x + 63/4 - 63/4 = 6x - 3 - 63/4

-x = 6x - 3 - 63/4

Subtract:

-x = 6x - 3 - 63/4

-x = 5x - 75/4

-x = 6x - 75/4

Subtract 6x from both sides

-x = 6x - 75/4

-x - 6x = 6x - 75/4 - 6x

Simplify:

-x - 6x = 6x - 75/4 - 6x

Combine:

-x - 6x = 6x - 75/4 - 6x

-7x = 6x - 75/4 - 6x

Combine:

-7x = 6x - 75/4 - 6x

-7x = -75/4

-7x = - 75/4

Divide by same factor:

-7x = - 75/4

-7x/-7 = -75/4 / -7

Simplify:

-7x / -7 = -75/4 / -7

Cancel terms that are in both the numerator and denominator:

-7x / -7 = -75/4 / -7

x = -75/4 / -7

Divide:

x = -75/4 / -7

x = 75/28

Thus the answer to your problem,
`(75)/(28)`