Question

F(x)=1,800(0.07)^x
f(x)=400

Answer 1

The solution for (x) in f(x) = 400 for the function
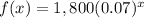`\(f(x) = 1,800(0.07)^x\)` is obtained by setting up and solving the equation, resulting in
`\(x = (\ln\left((2)/(9)\right))/(\ln(0.07))\)`.

The given function is
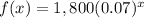`\(f(x) = 1,800(0.07)^x\)`, and you're seeking to find (x) when f(x) = 400. Set (f(x)) to 400 and solve for (x):

`\[400 = 1,800(0.07)^x\]`

To isolate (x), divide both sides by 1,800:

`\[(400)/(1,800) = (0.07)^x\]`

Simplify the fraction:

`\[(2)/(9) = (0.07)^x\]`

Take the natural logarithm (ln) of both sides:

`\[ \ln\left((2)/(9)\right) = x \cdot \ln(0.07) \]`

Solve for (x):

`\[ x = (\ln\left((2)/(9)\right))/(\ln(0.07)) \]`

Using a calculator, evaluate this expression to find the numerical value of (x).

In summary, the solution to f(x) = 400 for the given function
`f(x)=1,800(0.07)^x` is
`\(x = (\ln\left((2)/(9)\right))/(\ln(0.07))\)`.