Question

Suppose that Company A currently trades for $75.60 and the 3-month risk free rate is 4%. Using Put-Call Parity, what is the price of a three-month put option on Company A with a strike price of $80? A three-month call on Company A with a strike price of $80 trades for $3.91.

A. $9.12

B. $0.74

C. $0.31

D. $1.30

E. $7.51

Answer 1

Suppose that Company A currently trades for $75.60 and the 3-month risk free rate is 4%. The price of a three-month put option on Company A with a strike price of $80 is: E. $7.51.

What is the price?

Given data:

• Current stock price (S) = $75.60
• Risk-free rate (r) = 4%
• Call option price (C) = $3.91
• Strike price (X) = $80

Put-Call Parity formula:

`C - P = S - X * e^(-r * t)`

`P = C - S + X * e^(-r * t)`

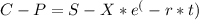
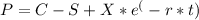
Plugging in the values:

P = $3.91 - $75.60 + $80 * e^(-0.04 * 3/12)

P = $3.91 - $75.60 + $80 * e^(-0.01)

P = $3.91 - $75.60 + $80 * 0.9900

P = $3.91 - $75.60 + $79.2

P = $3.91 + $79.2 - $75.60

P = $7.51

Therefore the price of a three-month put option on Company A with a strike price of $80 is: E. $7.51.