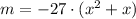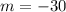Answer:
The value of
 is -30.
is -30.
Explanation:
Let
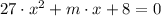 , we notice that expression is a second order polynomials. We can rearrange the polynomial and use factorization to calculate all roots:
, we notice that expression is a second order polynomials. We can rearrange the polynomial and use factorization to calculate all roots:
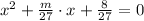 (1)
(1)
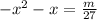 (2)
(2)
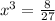 (3)
(3)
From (3) we find the least root:
![x = \sqrt [3]{(8)/(27)}](https://img.qammunity.org/2022/formulas/mathematics/high-school/loee6182cj68x5shtmk1dshkhhnsp2hqk8.png)
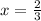
By (2) we have the value of
 :
: