It will take Charla
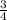 hour or
hour or
 minutes to complete the entire picture.
minutes to complete the entire picture.
Let
 represent the total time (in hours) it takes Charla to complete the entire picture.
represent the total time (in hours) it takes Charla to complete the entire picture.
She completes
 of the area in
of the area in
 hour.
hour.
The rate at which she completes the picture is given by the ratio of the completed area to the time:
![\[ \text{Rate} = \frac{\text{Completed Area}}{\text{Time}} \]](https://img.qammunity.org/2024/formulas/mathematics/college/1sojv94fbjc3qxbjaav5d0dy8oz5qwvsh4.png)
For the partial completion, the rate is:
![\[ \text{Rate}_{\text{partial}} = ((2)/(3))/((1)/(2)) \]](https://img.qammunity.org/2024/formulas/mathematics/college/cwl3n08ens29f8s78gr0sqn3womoziyh4r.png)
Now, for the entire completion, the rate should remain the same:
![\[ \text{Rate}_{\text{total}} = (1)/(t) \]](https://img.qammunity.org/2024/formulas/mathematics/college/2041eqsxt5sc8fjdiur0uzoae6mh9sfl2s.png)
Setting the rates equal to each other:
![\[ ((2)/(3))/((1)/(2)) = (1)/(t) \]](https://img.qammunity.org/2024/formulas/mathematics/college/cbqedfbqdv70go9p7y8gr2uzg2f7j3dmsw.png)
Simplify the left side:
![\[ (2)/(3) * (2)/(1) = (4)/(3) \]](https://img.qammunity.org/2024/formulas/mathematics/college/p361bs53n99ggnd7xcqo8g3ys583xt70p9.png)
So, the equation becomes:
![\[ (4)/(3) = (1)/(t) \]](https://img.qammunity.org/2024/formulas/mathematics/college/113jrpv0pwsk10zabqbcxiygwqijl1r92j.png)
Now, solve for
 :
:
![\[ t = (3)/(4) \]](https://img.qammunity.org/2024/formulas/mathematics/college/bnkriub1ayp7vfgnsqd7bcbl6ie9e2gh0q.png)
Therefore, it will take Charla
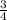 hour or
hour or
 minutes to complete the entire picture.
minutes to complete the entire picture.
The probable question may be: "charla wants to cover a certain rectangular area of her driveway with a picture using chalk. if she completes 2/3 of the area in 1/2 hour, how long will it take her to complete the entire picture?"