Question

The population of a town declined from 351,000 in 2010 to 333,000 in 2017. Assume the population decreases according to an exponential decay model.

a) Find the exponential decay rate, and write an exponential function that represents the population of the town t years after 2010.
b) Estimate the population of the town in 2022
c) In what year will the population reach 323,000?
a) Find the exponential decay rate.
k=
(Do not round until the final answer. Then round to the nearest thousandth as needed.)
Write an exponential function that represents the population of the town t years after 2010.
P()=
(Type your answer using exponential notation. Use integers or decimals for any numbers in the equation. Do not round until the final answer. Then round to the nearest
thousandth as needed)
b) In 2022, the population of the town will be about
(Do not round until the final answer. Then round to the nearest thousand as needed.)
c) The population of the town will reach 323,000 in the year
(Do not round until the final answer. Then round to the nearest year as needed.)

Answer 1

a) Exponential decay rate k : k = -0.017 , Exponential function:
`\( P(t) = 351,000 \cdot e^(-0.017t) \)`

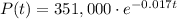
b) Population in 2022:
`\( \approx 335,000 \)`

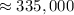
c) Population reaches 323,000 in the year 2024.

a) To find the exponential decay rate (k), we can use the formula for exponential decay:

`\[ P(t) = P_0 \cdot e^(kt) \]`

where:

- P(t) is the population at time t ,

- P_0 is the initial population,

- k is the decay rate,

- e is the base of the natural logarithm.

Given that
`\( P_0 = 351,000 \)` in 2010 and
`\( P(7) = 333,000 \)` in 2017, we can set up the equation:

`\[ 333,000 = 351,000 \cdot e^(7k) \`

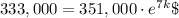
Now, solve for k :

`\[ e^(7k) = (333,000)/(351,000) \]`

`\[ 7k = \ln\left((333,000)/(351,000)\right) \]`

`\[ k = (\ln\left((333,000)/(351,000)\right))/(7) \]`

Now, calculate k to find the exponential decay rate.

b) The exponential decay function is:

`\[ P(t) = 351,000 \cdot e^(kt) \]`

To estimate the population in 2022
`(\( t = 2022 - 2010 = 12 \)` years after 2010), substitute
`\( t = 12 \)` into the equation and calculate P(12) .

c) To find the year when the population will reach 323,000, set P(t) to 323,000 and solve for t in the exponential decay equation.

Write the final answers rounding to the nearest thousandth for k , the nearest thousand for the population in 2022, and the nearest year for the year the population reaches 323,000.