Question

What are the roots of the equation 4x^2+12x+13=0 in simplest a+bi form?

Answer 1

Answer: x = -1.5 + i and x = -1.5 - i

Work Shown

There are two approaches. One is completing the square, and the other is the quadratic formula. I'll use the 2nd option.

Plug a = 4, b = 12, c = 13 into the quadratic formula.

`\text{x} = (-b\pm√(b^2-4ac))/(2a)\\\\\text{x} = (-12\pm√((12)^2-4(4)(13)))/(2(4))\\\\\text{x} = (-12\pm√(144 - 208))/(8)\\\\\text{x} = (-12\pm√(-64))/(8)\\\\\text{x} = (-12\pm8i)/(8)\\\\\text{x} = (-12+8i)/(8) \ \text{ or } \ \text{x} = (-12-8i)/(8)\\\\\text{x} = (-12)/(8)+(8i)/(8) \ \text{ or } \ \text{x} = (-12)/(8)-(8i)/(8)\\\\\text{x} = -1.5+i \ \text{ or } \ \text{x} = -1.5-i\\\\`

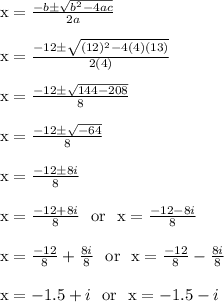
Notes:

• The two roots are of the form a+bi and a-bi where a = -1.5 = -3/2 and b = 1.
• I figured to use the decimal form, rather than fraction, but be sure to follow any instructions your teacher provides.
• The discriminant is negative (-64), which tells us we're dealing with two complex roots.
• 
  `i = √(-1)`
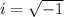
• I used the Csolve command in GeoGebra to verify the answers. This is slightly different from the regular Solve command because Csolve handles complex numbers of the form a+bi. Using the regular Solve command will lead to "no solutions" (since this quadratic doesn't have real numbers as roots).
• WolframAlpha is another option to check the answer. There are many online calculators.