Question

Find the missing side length. Round to the nearest tenth.

Can someone PLEASE HELP!!

Answer 1

the answer is 17.0 or just 17

Explanation:

you have to use the pythagorean theorem, a^2 + b^2 = c^2

a = 11 and b = 13

11^2 + 13^2 = c^2

121 + 169 = c^2

290 = c^2

square root each side, so you have to find the square root of 290 which is 17.0293864

round it to the nearest tenth which is 17.0 but you can just write 17

hope this helps you understand it and answer it :)

Answer 2

`\boxed {\boxed {\sf c \approx 17.0}}`

Explanation:

This is a right triangle because of the small square in the corner. Therefore, we can use the Pythagorean Theorem.

`a^2+b^2=c^2`

Where a and b are the legs and c is the hypotenuse.

In this triangle, 11 and 13 are the legs because they make up the right angle. The missing side is the hypotenuse because it is opposite the right angle.

`a=11\\b= 13`

`(11)^2+(13)^2=c^2`

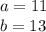
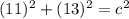
Solve the exponents.

• 11²= 11*11=121
• 13²= 13*13=169

`121+169=c^2`

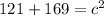
Add.

`289=c^2`

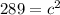
We are trying to solve for c, so we must isolate the variable. It is being squared. The inverse of a square is the square root, so take the square root of both sides.

`\sqrt {289}= \sqrt {c^2}`

`17.0293864=c`

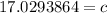
Round to the nearest tenth. The 2 in the hundredth place tells us to leave the 0 in the tenth place.

`17.0 \approx c`

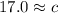
The missing side is approximately 17.0