Question

A model rocket is shot up at 50 m/s and at an of 70° with the horizontal. Which equation would allow you to find the

maximum height reached by the rocket?

Answer 1

The maximum height reached by the rocket is approximately
`\(113.67 \, \text{m}\)`.

To find the maximum height reached by the rocket, you can use the following kinematic equation for vertical motion:

`\[ h = (v_i^2 \sin^2(\theta))/(2g) \]`

Where:

-
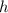`\( h \)` is the maximum height,

-
`\( v_i \)` is the initial velocity of the rocket (50 m/s),

-
`\( \theta \)` is the launch angle with respect to the horizontal (70°),

-
`\( g \)` is the acceleration due to gravity (approximately 9.8 m/s²).

Now, let's substitute the known values into the equation and calculate the maximum height:

`\[ h = \frac{(50 \, \text{m/s})^2 \cdot \sin^2(70\textdegree)}{2 \cdot 9.8 \, \text{m/s}^2} \]`

`\[ h = (2500 \cdot \sin^2(70\textdegree))/(19.6) \]`

`\[ h \approx (2500 \cdot 0.943^2)/(19.6) \]`

`\[ h \approx (2500 \cdot 0.889)/(19.6) \]`

`\[ h \approx (2222.5)/(19.6) \]`

`\[ h \approx 113.67 \, \text{m} \]`

Therefore, the maximum height reached by the rocket is approximately
`\(113.67 \, \text{m}\)`.