The half-life of the decay process is approximately 119 seconds.
To determine the beginning concentration of H2O2 and the half-life of its decay, we can use the second-order rate equation:
Rate = k[H2O2]^2
Given:
- Rate constant (k) = 5.32 x
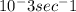
- Concentration of H2O2 after 320 seconds = 0.442 M
1. Calculate the initial concentration of H2O2 ( [H2O2]0 ):
To find the initial concentration of H2O2, we need to rearrange the rate equation:
Rate =
![k[H2O2]^2](https://img.qammunity.org/2024/formulas/chemistry/college/fbqa14d3hgteh93dqku730aynlzvawq2c4.png)
k = Rate /
![[H2O2]^2](https://img.qammunity.org/2024/formulas/chemistry/college/7y5x8ewmsh0d883d167zko4rdxxif102y4.png)
[H2O2]0 = (
 )
)
[H2O2]0 = √(0.442 M / (5.32 x
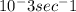 ))
))
[H2O2]0 = √(0.442 M / 5.32 x
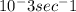 )
)
[H2O2]0 ≈ 16.62 M
Therefore, the beginning concentration of H2O2 is approximately 16.62 M.
2. Calculate the half-life:
The half-life (t1/2) is the time it takes for the concentration of H2O2 to reduce by half. In a second-order reaction, the relationship between half-life and initial concentration is given by:
t1/2 = 1 / (k[H2O2]0)
t1/2 = 1 / ((5.32 x
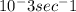 ) * (16.62 M))
) * (16.62 M))
t1/2 ≈ 1.19 x
 seconds
seconds
Therefore, the half-life of the decay process is approximately 119 seconds.