a. The discount price of the guitar is 31.50 (45 * 0.30).
b. If the regular price of an item is P dollars, the discount price is (0.70 * P) dollars.
c. The regular price of the trumpet is 200 (140 / 0.70).
a. The discount price of the guitar can be calculated by multiplying the regular price by the discount percentage:
Discount Price = Regular Price - (Regular Price * Discount Percentage)
In this case:
Regular Price = 45
Discount Percentage = 30%
Discount Price = 45 - 45 * 0.30
= 45 - 13.50
= 31.50
So, the discount price of the guitar is 31.50.
b.If the regular price of an item is P dollars and there is a 30% discount, the discount price (D) can be calculated using the formula:
![\[ D = P - (P * 0.30) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/t7t8q8twdzdpee6esfh6ghq1f6llnellky.png)
This formula represents the original price minus 30% of the original price, which gives the discounted amount. Let's break down the steps:
1. Set up the formula:
![\[ D = P - (P * 0.30) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/t7t8q8twdzdpee6esfh6ghq1f6llnellky.png)
2. Factor out P from the right side:
![\[ D = P * (1 - 0.30) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/vpfahbgi56v67nzadfrpv0xedkqvv25zy6.png)
3. Calculate the discount percentage:
![\[ D = P * 0.70 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/38c2l6babkdxyu69w361p2cbiucu7wtn09.png)
So, the discount price D is 70% of the regular price
 . If P is the regular price, then D is the discounted price after a 30% reduction.
. If P is the regular price, then D is the discounted price after a 30% reduction.
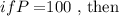
![\[ D = $100 * 0.70 = $70 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/wemq4i4517wn28cqamcvo1o5ztaq26ygv2.png)
Therefore, the discount price in dollars is 70% of the regular price.
c. If the sale price of a trumpet is 140, and we want to find the regular price P , we can rearrange the formula:
![\[ P = (D)/(1 - 0.30) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/2swmpamcfvxdfogp1u9rlbxigl6wguewj4.png)
Substitute
 into the formula:
into the formula:
![\[ P = (140)/(1 - 0.30) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/h7gmzyp2d46g5wcry7pcg1d47uzqtcpd1j.png)
![\[ P = (140)/(0.70) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/rwq6z9gi4sihy2arxb7w1mm68ga92yha4n.png)
![\[ P = 200 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/girzvrjwrq5htdehuz91c8fz4bdrz52baz.png)
So, the regular price of the trumpet is $200.