Question

Write the inequality shown by the graph.

The graph with X-coordinate marks -4, 0, and 4; Y-coordinate marks -4, 0, 4and 8. There is a line which passes through point (-6, 4). This line intersects y-axis at point (0, 2). There is shaded region which above the line.

Answer 1

The inequality representing the shaded region is:

y>− 1/3 x+2

From the information you provided, we know the line passes through points (-6, 4) and (0, 2). First, let's find the equation of the line using the two points:

The slope of the line (m) can be calculated using the formula:

m= y_2-y_1/ x_2 -x_1

​Using the points (-6, 4) and (0, 2):

m= 2−4/0−(−6)​ = −2​/6=-1/3

​Now that we have the slope (m) and one point (0, 2), we can use the point-slope form of a linear equation to find the equation of the line:

`(y- y_1​) =m(x-x_1 )`

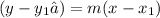
Using the point (0, 2):

y−2=− 1/3 (x−0)

y−2=− 1/3

y=− 1/3 x+2

This equation represents the line that intersects the y-axis at (0, 2). Now, the shaded region is described as being above this line. Since it's above the line, the inequality should indicate that y is greater than the line's equation. Therefore, the inequality representing the shaded region is:

y>− 1/3 x+2