Answer: The acute angle ∠YXZ = 24.3°.
Explanation:
If in triangle ABC, C is the angle included between sides a and b then
Area of triangle =
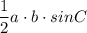
here, XZ = 21.5cm and XY = 13.5cm are sides that includes acute angle ∠YXZ..
Area of a triangle = 59.7cm²
then, as per given, we have

Hence, the acute angle ∠YXZ = 24.3°.